Introduction
A number is always the measured value when measuring a physical quantity. Using this number only makes sense if the corresponding unit has been given. A measurement’s outcome has a numerical value and an associated unit of measure.
Consider the weight of a human body, which weighs three kilograms. In this situation, employing a quantity with the numerical value three and the unit of measurement kg is necessary. It is the magnitude of a numerical value combined with the unit. Only the size of some physical quantities is necessary for a thorough description of the amount. Examples of the magnitude include body mass, the distance between two places, time, temperature, height, pendulum swings, and the number of books in a bag. In terms of direction, they are entirely adrift.
A scalar quantity is a quantity that just requires the magnitude of a quantity to be specified thoroughly.
Unit Vector
Unit vectors, represented by the symbol a, have a magnitude of one and are denoted by symbol 1. Unit vectors have a length of one. It is common to use unit vectors to express the direction of a vector.
What is the magnitude of a Vector?
Magnitude is the numerical value of any physical quantity, such as the distance between two points on a line.
The magnitude of a vector formula provides the numerical value for a given vector. We usually think of their direction and magnitude when we talk about vectors. The resultant of the various measurements of a vector along the x-, y-, and z-axes is represented by the magnitude of a vector formula. Vectors with negative magnitudes are denoted by the symbol |A|. Obtaining the magnitude of a vector having components along the X-axis, the Y-axis, and the Z-axis may be accomplished by computing the square root of the sum of squares of the vector’s direction ratios for each axis in the vector.
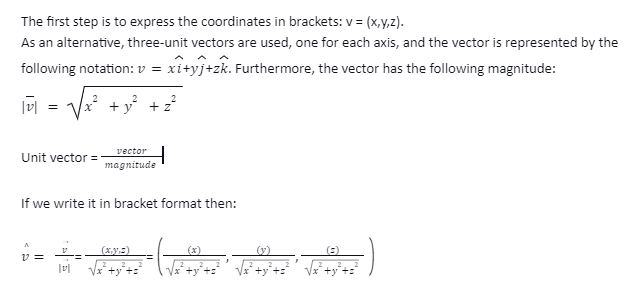
Unit Vector Formula
In this case, the vector is denoted by the letter a. Unit vectors also have a magnitude of one and are denoted by “^” and the letter a, which stands for “one unit vector.” A unit vector may be created by dividing any vector by the magnitude of the vector. This is known as the unit vector transformation. Furthermore, they are frequently expressed as XYZ coordinates. Again, there are two methods to go about it.
Unit Tangent Vector
Assuming v(t) is a smooth vector-valued function with a zero-threshold, any vector that is parallel to V‘(t) is considered to be tangent to the graph of v(t) at the time of the zeroth degree. It is most commonly used to represent the direction V’ (t) rather than the magnitude of this same direction.
We are thus examining the unit vector pointing in V ‘(t) direction. In this case, the following is the unit tangent vector definition:
Examine the smooth function V’ (t) on the open interval I, which is defined as follows: Thus, this example denotes the unit tangent vector as t(t).
t(t) =V‘(t)/|V‘(t)|
Unit Normal Vector
For simplicity, consider the vector-valued function V (t), in which the unit tangent vector, denoted by the symbol t‘(t), is smooth on the open interval. N (t) is defined as the unit normal vector n (t).
n(t) =t‘(t)/|t‘(t)|
The primary unit vector is another name for this vector.
Applications of Unit Vector
- In Physics, a unit vector is a vector that represents one unit.
- It is a vector in physics with an equal magnitude and a certain direction known as the unit vector. The sole direction is determined by a unit vector, the smallest vector. Dimensions and units are not provided for them.
- The x-axis, y-axis, and z-axis are all represented in a rectangular coordinate system.
- They are parallel and perpendicular to the other unit vectors in different coordinate axes.
Characteristics of Vectors
- It is necessary to comprehend their properties to grasp vectors and execute various computations utilising vectors.
- The dot product of two vectors is a scalar that resides in the plane of the two vectors used in the calculation.
- Crossing two vectors results in a vector perpendicular to the plane in which these two vectors are located.
Points to remember about Unit Vector
- Orthogonal unit vectors are always orthogonal to one another, and their dot product is always zero.
- It is always true that the cross product of parallel unit vectors is zero.
- When the cross product of two or more unit vectors equals zero, they are collinear.
- A non-negative number reflects the magnitude of the vector.
Summary
A vector is a quantity that possesses both magnitude and direction. A vector may be described as a line segment with a specific direction and a particular length from a geometric perspective. Scalars are physical values with just magnitude, such as mass, length, time, temperature, and the distance between two locations. Scalars are used in mathematics to represent the magnitude of physical quantities. It is convenient to portray a vector as a straight line with an arrowhead.