When describing gravitational force, the terms G and g are commonly utilized. Despite the fact that the acceleration due to gravity is tiny g, the universal gravitational constant is large G. Any object’s acceleration owing to gravity is represented by a small g. The universal gravitational constant is known as big G. It has a constant value of 6.67408×10_11 m3kg_1s_2and is a constant value. It has a unit of m3kg-1s-2 and is a constant value that does not change from object to object.
Acceleration due to gravity (g)
Any object’s acceleration owing to gravity is represented by a small g. This is normally reserved for huge things because small objects have very little gravitational force. Small g refers to the rate of change in velocity caused by gravitational force. This is a sort of acceleration that is only caused by gravity. This is a unit of metres per second squared (ms2) since it is an acceleration. The acceleration that an object receives when dropped is related to the earth’s gravitational pull. and we can call this acceleration “gravitational acceleration.” For the planet Earth, g equals9.8m/s2. Based on the mass and size of the object, it differs for different objects.
Universal gravitational constant (G)
The universal gravitational constant is known as big G. It has a constant value of of 6.67408×10_11 m3kg_1s_2 and is a constant value. It has a unit of m3kg-1s-2and is a constant value that does not change from object to object.
Relation between g and G on planet earth
The relation between g and G On planet earth is given as:
g=Gm1/r2
We could get the acceleration due to gravity on earth as 9.8 m/s2 by plugging in the values of m1and r for the earth. The radius at the equator is bigger than the radius at the poles, making our planet earth an ellipsoid. The value of g near the equator is greater than the value of g at the poles because gravity’s acceleration is inversely proportional to the square of the distance. The value of g changes from around 9.78 to 9.8m/s2near the Equator to approximately 9.83 to 9.8 m/s2in the poles, which is a relatively small variance. The value of G, on the other hand, is thought to be constant throughout the cosmos.
Relation between g and G with diagram
When a body is at rest on the Earth’s surface, it is affected by the Earth’s gravitational force. Let’s look at two methods for calculating the size of this force. Let M denote the Earth’s mass and m denote the mass of the body. The earth’s whole mass is thought to be concentrated at its centre. The earth’s radius is R=6378 roughly (6400km)The force acting upon the object is given by Newton’s law of gravitation.
F=GMm/R2……. (1)
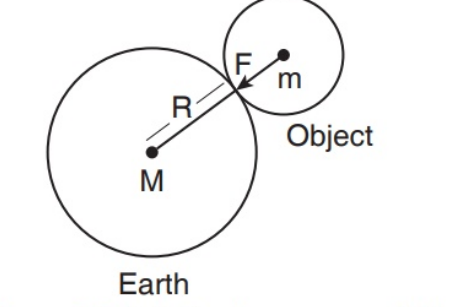
Relation between G and g is given by the above diagram.
When compared to the radius of the Earth, the radius of the body under consideration is insignificant. Newton’s second law of motion can now be used to calculate the same force. The force acting on a body is determined by the product of its mass and acceleration, according to this law (called as weight). Because the body’s acceleration is governed by gravity, a=g is used.
F=ma=mg…… (2)
Now comparing eq1 and eq2 we get :
mg= GMm/R2
Now Acceleration due to gravity is given as:
g= GM/R2
Conclusion
Gravitation is proportionate to the mass product and inversely proportional to the square of the distance between the masses. Gravity is defined as the product of a body’s mass and its continuous gravitational acceleration. Gravity is the gravitational force exerted by the earth. A body’s weight can fluctuate, but its mass remains constant. The acceleration owing to gravity is small g, yet the universal gravitational constant is big G. When a body is at rest on the earth’s surface, it is affected by the earth’s gravitational force.