A ray, segment, or line that divides a given angle into two equal angles is known as an angle bisector. The term bisector describes the division of a single object into two equal halves. A line or ray is used to divide a triangle and an angle in geometry, and this is known as an angle bisector.
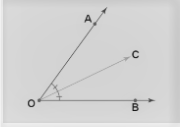
In geometry, the angle bisector is the ray, line, or segment that divides an angle into two equal pieces. An angle bisector, for example, will bisect a 90-degree angle into two 45-degree angles. It divides an angle into two smaller congruent angles, in other words. An image of AOB’s angle bisector is shown below.

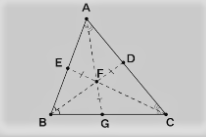
One angle bisector is a straight line that divides an angle into two equal or congruent angles in a triangle. Every triangle basically has three angle bisectors that are present in each vertex. The incenter of a triangle is the place where these three angle bisectors meet. The distance between the incenter and all of a triangle’s vertices is the same. Consider the figure below, which depicts a triangle’s angle bisector. The angle bisectors of ∠BAC, ∠ACB, and ∠ABC, respectively, are AG, CE, and BD. F is the incenter, or point of intersection, of all three bisectors, and it is at an equal distance from each vertex.

Theorem:
Consider the ABC triangle. At a point D between B and C, let the angle bisector of angle A intersect side BC. According to the angle bisector theorem, the length of line segment BD divided by the length of segment CD equals the length of side AB divided by the length of side AC.
BD/CD = AB/AC
If a point D on the triangle ABC’s side BC splits BC in the same proportion as the sides AB and AC, then AD is the angle bisector of angle ∠A.
According to the generalised angle bisector theorem, if D is on the line BC, then
BD/CD = ABsin ∠DAB / ACsin ∠DAC.
If AD is the bisector of ∠BAC, this reduces to the prior form. Directed line segments and directed angles must be employed in the computation when D is external to the segment BC.
When angle bisectors and side lengths are known, the angle bisector theorem is often applied.
The angle bisector of the vertex angle of an isosceles triangle will also bisect the opposite side as a result of the theorem.
Properties of an angle bisector:
You should now understand what angle bisector means in geometry. Let’s look at some of the qualities of the angle bisector, which are stated below:
An angle bisector is a tool that divides an angle into two equal halves.
Any point on the bisector of an angle is equidistant from its sides or arms.
It divides the opposing side of a triangle by the ratio of the measurements of the other two sides.
Construction of angle bisector:
Let’s try making the angle bisector for a given angle. The steps for constructing an angle bisector are outlined in this section.
Construction of an Angle Bisector:
Step 1: Draw any angle you want, such as ∠ABC.
Step 2: Draw an arc with B as the centre and any relevant radius to cross the rays BA and BC at, say, E and D. (See the diagram below.)

Step 3: Draw two arcs to intersect each other at F, using D and E as centres and the same radius as in the previous step.
Step 4: Make a ray by connecting B and F. The needed angle bisector of angle ABC is ray BF.
Conclusion:
The angle bisector theorem is a geometric concept that deals with the lengths of the two segments that a line bisects the opposite angle divide a triangle’s side into. A ray, segment, or line that divides a given angle into two equal angles is known as an angle bisector. The term bisector describes the division of a single object into two equal halves.
In geometry, the angle bisector is the ray, line, or segment that divides an angle into two equal pieces. An angle bisector, for example, will bisect a 90-degree angle into two 45-degree angles.
Properties of angle bisectors are, An angle bisector is a tool that divides an angle into two equal halves. Any point on an angle’s bisector is at an equal distance from the angle’s sides.
When angle bisectors and side lengths are known, the angle bisector theorem is often applied. The angle bisector of the vertex angle of an isosceles triangle will also bisect the opposite side as a result of the theorem.