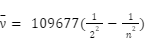
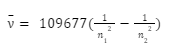
Lorem ipsum dolor sit amet, consectetur adipiscing elit. Ut elit tellus, luctus nec ullamcorper mattis, pulvinar dapibus leo.
Introduction:
Emission of radiation occurs when electrons return to their former states after absorbing energy and becoming excited, which causes them to jump from lower energy levels to higher energy levels. A well-known truth is that this is the case. This phenomenon explains the hydrogen emission spectrum, which is also called the hydrogen emission spectrum.
Using an electric discharge and a diffraction grating to study the light emitted from an excited gas, scientists discovered in the late 1800s that the resulting spectra did not consist of a continuous band but rather of discrete lines with clearly defined wavelengths. Scientists conducted experiments that revealed its unique wavelengths to better understand the chemical element that emitted the light. They were an atom’s interior structure that resulted in an atomic fingerprint.
Origins of the Hydrogen Emission Spectrum:
- It is possible to express the hydrogen emission spectrum in a (relatively) simple equation. Simple whole integers can be used to calculate each line. A high voltage causes a chemical reaction that results in the emission of light.
- Hydrogen’s electron is in the first energy level, which is closest to the nucleus when it is not excited. Electrons can be transported up or out of the atom depending on the amount of energy supplied to it. A discharge tube’s high voltage supplies such energy. Emitted electrons from hydrogen molecules are split apart and promoted to higher energy levels, which is why the atomic hydrogen emission spectrum is named after it.
- Let’s say an electron has been stimulated to its third energy level as an example. A drop in altitude would cause it to lose energy once more. It has two options for accomplishing this. Alternatively, it may return to the second level and then make a second jump back down, returning to the first level.
Assigning specific electron jumps to specific spectral lines:
The amount of energy an electron loses when it falls from the 3-level to the 2-level is equal to the difference in energy between those two levels. As the electron loses energy, it emits light (which “light” includes UV and IR as well as visible radiation). The equation below explains how each frequency of light is linked to a specific energy.
E = hv
Here h is Planck’s constant and v is the frequency of the radiation.
- Light’s energy rises in direct proportion to its frequency. Red light is produced when an electron falls from the third to the second level.
- This is where the hydrogen spectrum’s red line comes from.
- The energy of the red light can be determined by its frequency. The energy gap between the hydrogen atom’s 3-level and 2-level must be exactly the same.
The highest frequency line in the spectrum will consequently be produced by the biggest drop in energy conceivable. A fall from infinity to one is the steepest.
The wavelength of the hydrogen spectrum:
- Photon absorption leads electrons to transition to higher energy levels, for example, n = 1 or 2 for hydrogen.
- For example, when an electron in hydrogen has emitted a photon, the electron transitions from a higher energy level to a lower energy level (n = 3 to n = 2).
- Light is transmitted due to this descent from one level to another. These energy levels, quantized, are responsible for creating wavelengths of light that reflect these variations.
- For instance, the transition n = 3 n = 2 is shown by the line at 656 nm.
Hydrogen emission spectrum:
When Balmer proposed the equation for correlating the wave number of spectral lines and energy shells in 1885 based on experimental results, he revolutionised our understanding of hydrogen emissions. The following is the formula:
![]()
The Balmer series is a type of hydrogen emission spectrum. In the electromagnetic spectrum, this is the sole set of lines that can be seen. The value of 109,677 cm-1 is the Rydberg constant for hydrogen. The component of the hydrogen emission spectrum known as the Balmer series is responsible for the excitation of electrons in any shell other than the second. The series names for other transitions are similar. The following is a list of some of them.
- A transition from one shell to another: the Lyman series,
- A transition from 2nd shell to another: the Balmer series
- A transition from 3rd shell to another: the Paschen series,
- A transition from 4th shell to another: the Bracket series,
- A transition from 5th shell to another: the Pfund series.
For the estimation of the wave number of hydrogen spectral line emissions owing to the transit of an electron from one orbit to another, Swedish scientist Johannes Rydberg created a generic formula. The following is the general formula for calculating the hydrogen emission spectrum:
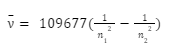
Where,
- n1 = 1, 2, 3, 4,…
- n2 = n1 +1
The hydrogen Rydberg constant has a value of 109,677 cm-1.
Conclusion:
Here are a few key takeaways from this learning:
- Evidence for an atom’s quantized electrical structure can be found in the hydrogen spectrum.
- When an electric discharge is applied to a gaseous hydrogen molecule, the hydrogen atoms of the molecule dissociate.
- As a result, energetically excited hydrogen atoms emit electromagnetic radiation. The hydrogen emission spectrum consists of a range of frequencies. The scientists that discovered these radiation series gave them their names.