An electric field is said to be a property that exists in the space where any charge is present. Another term that can be used to describe electric fields is force per unit charge.
The formula to calculate the electric field is:
E=F/Q
where, F is considered to be the electrostatic force and Q be the charge.
The events that cause an electric field are changing magnetic fields and electric charges. The volt per metre (V/m) is the SI unit for measuring electric field strength.
The direction of the electric field is given by the direction of the force acting on the test charge. Positive charge produces a radially outward electric field, while negative charge produces a radially inward electric field.
Electric field:
The electric field is technically described as a vector field that may be associated with each location in space and represents the force per unit charge exerted on a positive test charge at rest at that point.
The electric field is created by the electric charge or by magnetic fields that change over time. The electric field is responsible for the attraction forces that hold the atomic nucleus and electrons together at the atomic scale.
Let us assume a charged particle Q at position X and a test charge q at position x.
Now, the electrostatic force on the test charge will be given as:
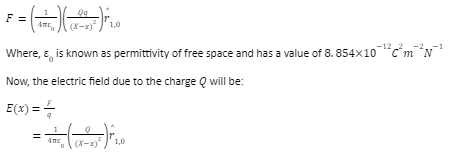
Electric Dipole:
When a pair of opposite charges let it be +q and-q are separated and the distance between them is d then this arrangement is known as electric dipole. The direction of an electric dipole is from the negatively charged particle towards the positively charged particle. The centre of the dipole is the point where the two charges meet. A pair of electric charges that have opposite signs but are equal in magnitude separated by distance is the simplest example of an electric dipole.
The symbol for an electric dipole is P
The product of the magnitude of the charges multiplied by the distance between them is known as an electric dipole, which can be expressed mathematically as P=Qd where Q is the magnitude of charges and d is the distance between the charges.
Direction of Dipole moment:
The electric dipole moment is a vector quantity whose direction is from the negative charge towards positive charge. It’s very important to remember, however, that this orientation norm is solely followed in Physics. But, in chemistry this convention is reversed, that is the direction will be from positive to negative. The axis of the dipole is the line that goes through the charges and the direction of the electric dipole
Significance of dipole:
The electric dipole is an important topic in both electrostatics and chemistry. Because the centres of positive and negative charges in most molecules occur at the same place, the distance between two charges can be considered as zero. Carbon dioxide and methane are said to lie in the zero-dipole moment category. This type of molecules that have zero dipole moment are known as non-polar molecules. Polar molecules are those that have a persistent dipole moment because the centres of positive and negative charge are at some distance.
Electric dipole used in Scientific application:
In the sphere of science, the electric dipole moment has a number of essential uses. When the influence of microscopic charge separation is obvious, but the real distance between these charges is too small to calculate, this is a helpful idea.
The majority of molecules have permanent electric dipole moments, as has been seen. When molecules and atoms are held in an external electric field, they can acquire a permanent electric dipole moment.
An “electric dipole moment” potential is calculated by summing the potentials of the positive and negative charges that generate it. In layman’s terms, an electric dipole moment is the accurate measurement of the strength associated with any electric dipole.
A study of the electric dipole can help you gain a better grasp of polar compounds’ applications and performance. These are molecules that have an electric dipole moment even when they are not in touch with an electric field. The mid-regions of a negative charge and a positive charge coincide in most molecules; therefore, their electric dipole moment is zero.
Conclusion:
A pair of opposite charges q and –q separated by a distance d is referred to as an electric dipole. The direction of the electric field is always defined from the negatively charged particle towards positively charged particle. The dipole’s centre is defined as the point where q and –q meet. A pair of electric charges which possess opposite charges and are separated by a distance is regarded as the simplest example of an electric dipole. In a uniform electric field, the net force acting on a dipole is zero. The forces acting on the dipole’s two charges are equal and opposite. As a result, the net force is null.