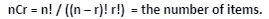

What is a Combination?
In permutation and combination, a combination is a mathematical method that focuses on determining the number of apparent treaties in a compilation of elements. An individual can choose the items quickly. In combination, the order of the choice is straightforward. Combinations often get disturbed with permutations. For instance, the configuration of ab and ba remain the same in combinations. Instead, it is considered one arrangement, whereas, in permutations, the structures are different. Nonetheless, in permutations, the selection method of an item is necessary. Combinations are surveyed in combinatorics but are expanded in several domains, including calculation and finance.
Combination Formula
Mathematically, the Combination Formula for inferring the number of apparent treaties by assigning a limited issue barely from a pair with no reduplication is conveyed in the following way:
n stands for the gross quantity of components in a bunch.
k stands for the number of selected issues (as the order of the objects is not essential)
! indicates factorial.
Factorial (noted as “!”) is a property of all optimistic numerals insufficient or comparable to the amount preceding the factorial evidence. For instance, 3! = 1 x 2 x 3 = 6.
Note that the formula above can be employed barely when the objects from a pair are appointed without reproduction.
Example of Combination
The following is about a manager’s portfolio in a minor hedge fund. The manager is supposed to develop a new fund to prevent risk-taking investors. The fund perhaps includes commodities of rapidly-growing firms offering increased outgrown capabilities. The analytic team specified the stock of closely 20 companies according to their profile. The manager further involved five additional supplies and proportional weight in the primary portfolio. It is just because the fund is new. At present, the manager desires to identify several probable portfolios. It will help him develop from those stocks identified by the analyst team.
It is a decision-making process in investment and the above example is about a combination problem. Since an individual will create a portfolio in which the entire stocks will be of the same weights. The order of the chosen stores will not affect the portfolio. For instance, the portfolio ABC and CBA will remain the same for each stock because of the same way.
Definition of Permutation and Combination
Instead, it is essential to understand permutation and combination better and the relationship and differences between them.
Most of the possibilities of mathematical permutation happen. In premutation, an individual arranges a data pair in a specific order or sequence. However, if the data is ultimately set in order, the individual has the power to rearrange them. In such cases, the individual will use the permutation formula. On the other hand, a combination for another, contrary to premutation, is when an individual selects data from a year without any order or even sequences. If the data group is comparatively lesser, an individual can measure the number of probable combinations. This definition is elaborated below with potential permutation and combination search examples.
For instance, an individual has a group of four letters P, Q, R, S. Now, and there are different ways an individual selects three letters from this group. Every possible arrangement can be a combination.
Permutation Formula
If the entire amount of data is “n” and the selection is of “r” things, accordingly permutation will be without alternate and respecting an order of

Conclusion
A combination is barely a way of appointing some objects from a provided pair of things in a specific way that the order of their choice doesn’t matter. Moreover, it is determined that one is not assigning a sole commodity further once, i.e. reduplication is not permitted. On the other hand, it is a combination. The order of objects is affordable. It is only the preference of valuable things, not its configuration with appreciation to other appointed entities.